4.03 Energy Equation: Constant Pressure

The energy equation of thermodynamics equates rate of system energy change to its causes which might be mass transfer, rate of work effects, friction and/or heat. System events occuring at constant pressure are common. Simple heating, cooling or phase change at one atmosphere are examples.

It is convenient to have a specialization of the rate-form energy equation for application to constant pressure events. Equation specialization is the saame for open or closed systems. Closed system is used here to mininmize writing.

The closed system energy equation written in its rate form is:

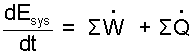
(1)

The form of Eqn-1 "implicit," meaning its terms are "symbolic" rather than detailed. To specialize Eqn-1 for constant pressure events, we identify system energy, E, compo­nents as being "internal" and/or "external." Internal energy is notated, U. The system "external" energies are its kinetic energy, KE, and potential energy, PE.

Consequently, we can rewrite system energy (Eqn-1 ~ left-of-equality), E, in terms of U and KE + PE.

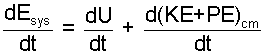
(2)

Syetem energy is internal, U
kinetic, KE and potential, PE

Returning to Eqn-1, the first term right of equality represents the sum of system "work rates." This term can be written as the sum of two components. The first component is "external," it is related to the system rate of "external energy" change, ΣW_KE,PE. The other component is related to the rate of "internal energy," change, dU/dt. Eqn-3 shows the original term written as its components.

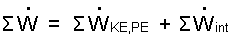
(3)

System work rate associates
with rates of KE and PE
and the rate of internal energy,
U and .

Next we substitute Eqn-2 and Eqn-3 into Eqn-1.

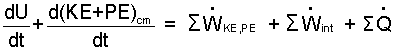
(4)

This is Eqn-1 expanded to
show detail of its terms.

Equations 1 and 4 are the rate-form energy equation. Eqn-4, however, is more "explicit." It shows a breakdown of terms, rearranged. The rearrangement permits the equation to be separated into "external" and "internal" components. The energy-equation, external part shown as Eqn-5.

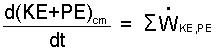
(5)

This is the "work-energy" 
equation of mechanics.

When Eqn-5 is subtracted from Eqn-4, what remains is the internal energy equation, Eqn-6.

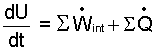
(6)

The "thermal" energy equation applies
when energies of motion and position
are irrelevant.

Some further specifications of Eqn-6 are needed. The types (or modes) of possible system work, W_int include spring work, elastic work, compression work, torsion work, surface work, electric work, electric polarization work, magnetic polarization work... individually or combinations thereof. Work might be complex but usually it is not.

The work mode all substances have is the "compression work," W_comp. These substances are said to be "simple compressible" and to experience "compression work." Eqn-7a presents "compression work." Compression work is expressed as Eqn-7a. Eqn-7b expresses "rate of compression work."

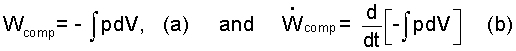
(7)

Compression work and
compression work rate.

When W_comp is substituted into Eqn-6 for ΣW_int for work rate, the energy equation becomes Eqn-8a. Or, in the rate form, Eqn-8b.

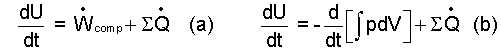
(8)

Algebra!

There is a simplification for constant pressure events. The initial term is below left, Eqn-8. Changes, from left-to-right yield the right-most form.

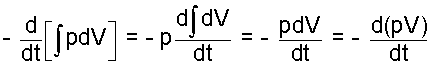
(9)

Here, the compression work term is
specialized for constant pres-
sure events.

Now place the result, Eqn-9 into Eqn-3... With a little more algebra:

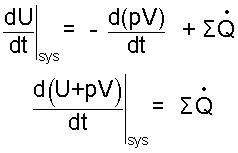
(10)

Here the term d(pV)/dt
is moved "left--of-equality
then combimed with dU/dt.

So by the above manipulations, something simple results.

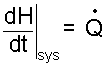
(11)

Enthalpy is a natural consequence, a valuable property for description of constant
pres­sure events. Enthalpy also arises for open system passage of matter across a
system boundary.