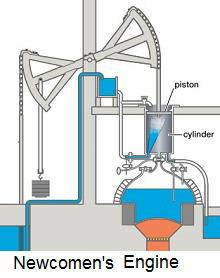
Atmospheric Engine

Thomas Newcomen invented this piston and cylinder engine in 1712. The power stroke of the atmopheric steam engine is an ingenious thermodynamic event. A fine antimation of the engine's operation has been coded. Animation URL!.

"How it works," is explained using three idealized instances: (i) Initial Condition, (ii) Water Injection, and lastly, (iii) Equilibration of the water and steam.

(i) Initial Condition: Briefly described, from a fired boiler, steam is piped into the cylinder bottom and out its top until all air is ejected and the cylinder wall and piston are "steam" hot. Next, as the piston rises from least to maximum height, steam at 100°C (g1) fills the maximum cylinder volume. Fitted to the large piston/cylinder is a smaller "injection" cylinder full of water (f1). Our system is the steam and water. Fig 1. depicts the situation.

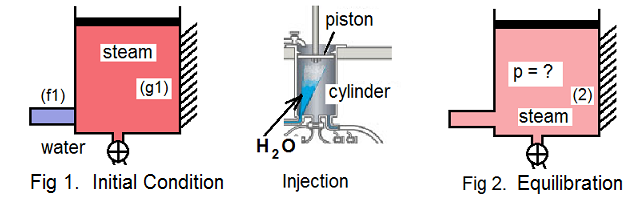
It is convenient to calculate properties of the Initial Condition now. Table 1. contains necessary assumptions and properties of water.

Table 1: Assumptions - Properties @ 1 atmosphere
Steam (g1) V_cyl = 1.000m³ T = 100°C v_g = 1.667m³/kg, u_g = 2506kJ/kg	Water (f1) V_inj = 0.001m³ T = 24°C v_f = 0.001m³/kg u_f = 100.8kJ/kg

Necomen's Engine requires the three equations, two physical principles: volume is constant and energy constant (Eqn-6), along with tabular properties for water be solved simultaneously. The system volume is that of the steam and injection water.

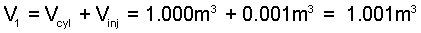(1)

The discrete masses of the system are determined via specific volumes and volumes.

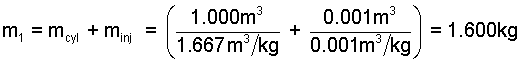(2)

The cylinder of steam saturated at one atmosphere and the injector water saturated at 24°C have internal energies. The system internal energy is:

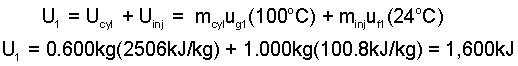(3)

#################

(ii) Injection: Into the steam in the sealed piston/cylinder is violently jetted the injection water. We assume this to occur instantly (hence no heat). The system mass is constant and while the piston can move, there is too little time for volume to change (hence no work). Also the internal energy remains what it was. For the injection event, mass, volume, and internal energy are constant.

#################

(iii) Equilibration: When water is sprayed into the hot steam, it will condense and the resulting pressure will be less than the original, 101.3kPa. Our interest is to prove the "result of quench" will be lower pressure. The thermodynamic event is called "equilibration," whereupon the initially separate steam and water transform from initial temperatures and one atmosphere to a same temperature and lower pressure. Equations must be written then used with water properties to prove this fact.

For equilibration, system volume is constant:

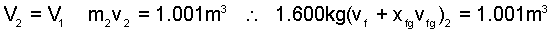(4)

For equilibration, system internal energy is constant:

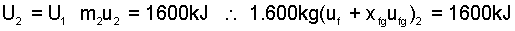(5)

#################

Below are "final forms" of Equation (4) and (5) algebraically arranged such that each has its second quality left of equality. These equations (6) apply to the "equilibated" or quenched state." Below Eqn-6, Table 2 is a brief listing of properties of water.

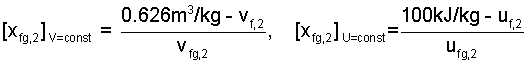(6)

Table 2: Saturation Properties for Water
p(kPa)	v_f(m³/kg)	v_fg(m³/kg)	u_f(kJ/kg)	u_fg(kJ/kg)
90	0.001	1.87	404.9	2097
60	0.001	2.73	359.7	2489

Necomen's Engine requires the three equations, two physical principles: volume is constant and energy constant (Eqn-6), along with tabular properties for water be solved simultaneously.

The two equations and table constitute a set that can be solved for the second temperature and pressure. But since the table is involved, we must use trial and error. So we...
   (i) Guess the equilibrated pressure, p*.
   (ii) Use Table 3. to obtain water properties: v_f(p*), v_fg(p*), u_f(p*), and u_fg(p*).
   (iii) When the properties of water at p* are substituted into Eqns-6 two values of x_fg(p*)    are obtained. If the two values x_fg(p*) are not equal - try again.

Table 3 presents trial pressures and the qualities (x_fg,2) associated for tries at 60kPa and 90kPa. We observe the "constant volume" curve and the "constant internal energy" to curve cross each other - indicating a solution of about 75kPa.

Table 3: Trial and Error Data
p(kPa)	[x_fg,2]_{V = const}	[x_fg,2]_{U = const}
90	0.33	0.28
60	0.23	0.26

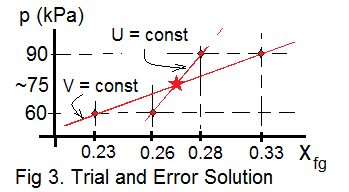
75kPa seems a pretty high pressure. We would like it to be lower. But this is our first, guessed case of the apparatus. This is not Necomen's solution. Further design might yield better performance. However, I'm tired of this now... Maybe I'll do more later!