| THERMO Spoken Here! ~ J. Pohl © | TOC NEXT ~ 70 |
2.03 Hydrostatic Equation
Let the physical scenario be the simple situation of an amount of fluid in a static condition above Earth. Locations within the fluid have the properties position, density and pressure. An equation, relating these properties is derived below. Without loss of generality, let the fluid be atmospheric air.
The equation to be derived is an extension of Newton's 2nd Law of Motion. In its development Newton applied his 2nd Law to a BODY as system (or subject of interest). as the subject or system. In this case Newton's Law will be applied to a ststic fluid.
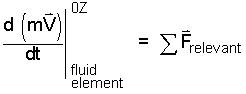
| (1) 1 |
To proceed, a system (a small amount of mass) is selected. Next, Newton "isolated" the system. This "analytic endeavor" is to "remove the system" from its surroundings entirely.
Generally a sketch is drawn that initially shows the system (amount of mass) set aside from the rest of physical reality, that part not the system is called surroundings. The purpose of isolation of the system is to observe (by means of analysis) the event of the system.
However, with the system now "set aside" from its surroundings we realize, nonetheless, that the surroundings might contribute some effect on the events. To allow for that possibility, to include the potentiality that surroundings might cause an event, we add to our analytic method a construct. That construct is called "force." Thus the completing steps of sketching our system are to graphically notate forces that are known to act and others that might be unknown.
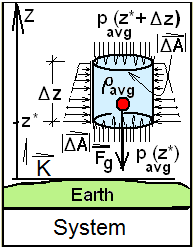
To continue, our system, taken from the fluid of the scenario, is shown as a selected and removed cylindrical volume of liquid. The surrounding liquid has been removed leaving a vertically-oriented right-circular cylinder of liquid (Sketch below ~center panel).
Units of the coordinate, Z, indicate elevations above Earth. The height of the cylinder is ΔZ, the cross-sectional areas of its ends are oriented horizontally, they measure ΔA in area.
When the fluid of the surroundings are removed from contact with the system at its surface, (the outer surface of our imaginary cylinder) small forces are drawn to represent what we call "fluid-pressure-forces," or the effect of fluid-pressure acting over small areas of the surface. Pressure-forces act over the entire boundary of our cylindrical element. (Those that act on the cylinder side act circumferentially around the cylinder. These are difficult to draw without cluttering the figure.) Also note that Earth is part of the surroundings. When the system is isolated, a gravity force is drawn on the sketch.
Usually the "system" is sketched without a sketch of the "scenario." We go one step further in our explanation. The right panel above shows the surroundings, that is, what is left of the "scenario" once the system is isolated from it. A hole in the fluid is left with pressure forces acting on its surface. As a last point, notice that were the "system sketch" and "surroundings sketch" brought together the result (with forces adding to zero) would be the original scenario.
Next we write the Momentum Equation for a Body (Newton's Second Law of Motion) applied to the cylindrical element. (Note: At the beginning of this development, the cylinder of fluid certainly does not qualify as matter at a point, as a BODY). We begin with the general form:
We require the momentum of the cylinder to be constant such that its derivative,""d/dt" equals zero. For this to be true, the element velocity need not be zero; it might be moving, but at a constant velocity. Therefore we set the derivative of momentum equal to zero (notated as a "cross-out," X). Also we expand the summation of forces to identify the gravity force, pressure force over the top, over the bottom and about the perimeter of the cylinder. As a last step, the equation is multiplied by the unit vector, K.

| (2) 2 |
All of the pressure-forces that act on the cylindrical part of the system surface are perpendicular to the K vector. Consequently those products with the unit vector equal zero. We assume we know the average pressure over the horizontal top and bottom of the cylinder. We write these as functions of their z-coordinates.
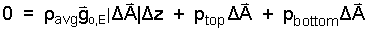
| (3) 3 |
Substitute the expressions for the pressures and do a touch of algebra to obtain:

| (4) 4 |

| (5) 5 |
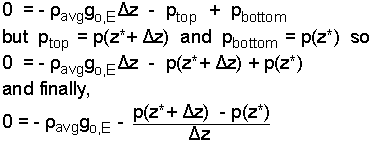
| (6) 6 |
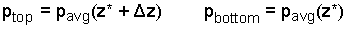
| (7) 7 |
To proceed past this point we need the ideas of Newton's Calculus. Specifically we apply a "limiting" operation to the entire equation. The notation for this operation is written as:
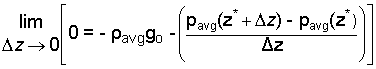
| (8) 8 |
The limit of the constant, ρgo is the constant itself.
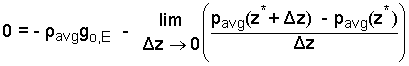
| (9) 9 |
Our mathematics has arrived at calculus. The term "right-most" in the equation above is (by the work of Newton and Leibnitz) the "limit" of a difference quotient. There is a special notation for that entity, a more compact way of writing it - the right-most term of the equation below.
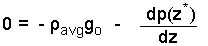
| (10) 10 |
When Δz shrinks to zero with ΔA also very small, the average density becomes the value at z = z*. Now we have carried this superscript star to indicate that the elevation was a value of z. (To have used simply z , is incorrect. The derivative is defined at a point Ask your math prof about this).
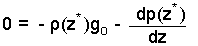
| (12) 12 |
The above is one idea away from our final result. The "point" we used to define the derivative was the location " z*" . That point was arbitrary; said otherwise, our results would be the same had any other location been selected. That being the case, any " z " could have been used. We drop the asterisk to have the variable become z.
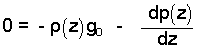
| (13) 13 |
This last equation is our result. The "zero" left of equality reminds us that the fluid element has constant momentum. The two terms right-of-equality are the consequence when the sum of forces is shrunk to a point. Although the above form has physical meaning, the equation is always altered for use. The following form is called the Hydrostatic Equation.

| (14) 14 |
This is a first order differential equation. Its solution requires specification of the fluid and a value of the pressure at one value of elevation. This chapter contains many example solutions.
2.03 Hydrostatic Equation

Consider the simple physical scenario of an expanse of air (a fluid) that exists above the Earth surface. Locations within the fluid have the properties position, temperature, density and pressure. An equation that relates there properties has been derived using Newton's method of system analysis. Below that derivation is presented and explained. Without loss of generality, let the fluid be atmospheric air.
Premise presently unwritted!