1.10 About f = mA

Typically, HS students are taught Newton's Laws of Motion by use of an algebra-based, "ab," approach. The idea is to simplify Newton's Laws so they are easier to understand. However, to formulate and write his Laws, Newton invented vectors and calculus. This was not to be fancy; Newton knew mathematics more powerful than algebra was needed. Now,some 450 years after Newton, HS physics still teaches his Laws "in first gear" unemcumbered by vectors or calculus.

Most physics texts present Newton's idea, his 2nd Law of Motion, as the words "force equals mass times acceleration." Students learn the idea, saying the words like a mantra: "f equals ma." To procede, texts assign symbols to force, mass and acceleration then represent the idea mathematically as an algebraic equation. Mathematics is the means whereby symbolic physical entities (force, mass, et al) are made quantative. The idea is abstract. Application will be

The words of the second law and its mantra are abstract, just beginnings of the idea. Text are alike to this point. Text for text the words and form of the equation are the same but the equations have different notations. Inspection of three texts yield these equation forms equal algebraically but with different notations.

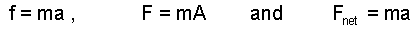
(1) HS text forms of Newton's 2nd Law of Motion.

Suppose we write Newton's 2nd Law in its HS algebra-based form then change it to use vectors (origin, basis, unit vectors...) then modify it to include concepts of calculus (difference, limit, derivative...). Changing backward toward what Newton wrote in 1687, step by step, back in history. What would the original 2nd Law, before the ink was dry, look like. Also, with this task might we find a superior notational form for the algebraic-based 2nd Law? If so, what is it?

Newton's writings regarding the Laws of Motion express his emphasis. Precedeing his laws he wrote two axioms and he specified a physical model for analysis.

====

System modeled as BODY:  The subject (system) of Newton's Laws are a "model" of physical reality, the "BODY." This simplification of the mass of something physically real to be idealized as mass located "at a point."

Axiom II:  "...quantity of motion" Newton defined "quantity of motion" to be a measurable, scalar times vector, mass times velocity.

BODY:  ... real mass assumed to exist at a point.

Axiom II:  "...quantity of motion" today is called "momentum." Momentum is a vector-calculus idea. The momentum of a mass requiores special specification. Momentum for a BODY is written as:

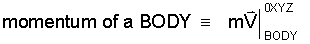
Newton's Three Laws:  The names, "First, Second..." cause one to rank the ideas.

Newton's First Law:  in his statement... "Every BODY..." Body is a model of physical reality. While any real amount of mass (a body, as we might say) occupies space (has a volume) Newton's perspective of mass was that it had no volume. The subject of the 2nd Law is an amount of physical reality which has a mass. To generalize, let's call that selected mass the system. Newton used the very simplest model of system - the BODY; all mass located at a point.

The 2nd Law addresses an aspect of that mass; its momentum (m_BODYV_BODY). By his second axiom, Newton stated that "quantity of motion" (or momentum as called today) was the property of motion of a BODY.

The 2nd Law of Motion (with momentum, "mV," as the independent variable) is a first-order differential equation. Newton did not use mathematics "to be fancy."

In most HS physics texts, Newton's motion, his idea "momentum," (the vector entity, mass times velocity product) is replaced by the scalar product, mass times acceleration.

Many physics-text-forms of Newton's Second Law have F or f written left-of-equality. In the paragraph immediately beneath the equation it is stated that F and f do not represent a force. Rather F or f represent a vector sum of all forces applicable. Some texts use the notation F_net which is a vector sum of all forces applicable.

The mantra taught is "force equals mass times acceleration."

Most applications of Newton's 2'nd Law involve more than one force. There is a common mathematical notation to designate an equation term as being the "discrete sum of its occurrences." That notation is to prefix the term with the Greek upper-case letter sigma, Σ. When force, F, is prefixed with "ΣF," the meaning is clear: "this term is the sum of all relevant forces - be sure to identify and sum the forces."

We now return to equations (1) and rearrange as Equation (2)(below left):

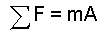
(2) The commonly used, F, is in fact a ΣF.

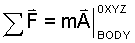(3) It is motion of a “BODY” we observe. Vectors (position, velocity, force) are written in the “0XYZ” vector space.

We choose to make Equation (2) more specific, to become Equation (3). Forces and acceleration are not algebraic entities; they are vectors. The distinction, what is a scalar versus what is a vector is important. Vector entities are written (here) with an over-arrow (and sometimes with an over-bar). To specify a vector, a vector space, origin, coordinate axes, and a unit vector basis, must be defined. Students are familiar with Cartesian coordinates (0XYZ). Around 1850, Sir William Hamilton invented the unit vector triple, I, J and K. Since equations contain thought, equations with vectors should identify their space. (To identify space is a necessary skill for those who program video-games or the actions of robots).

"mV," is called its "momentum."

Finally, about Equation (3), the system of Newton's Laws was a collection of matter he modeled as a BODY (the simplest approximation of matter). Specifically the mass and acceleration of equation (3) are those of the BODY. We place that subscript behind those terms then move them left-of-equality for reasons explained below.

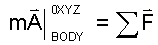
(4) System (with its system states) is left-of-equality. The actions of Forces (right-of-equality) might change system states:

Newton invented calculus not for fun but to define velocity and to define acceleration, the A of f = ma.

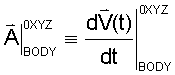
(5) The “left” term is identically the same as the “right” term. The equation simply identifies a “shorter” way of writing things"

Acceleration is a characteristic of the motion of something (we call it a BODY) in space (we use the Cartesian space, 0XYZ). Acceleration equals the derivative of the velocity of the something in the space (written above left).

Below Left: mass of a BODY is multiplied by the acceleration of that BODY. Acceleration is the derivative of velocity. Therefore (as shown left) mA = mdV/dt. Below Right: the mass term (a constant) is brought inside of the derivative ("d/dt") showing that mA = d(mV)/dt which is our preferred form of mA.

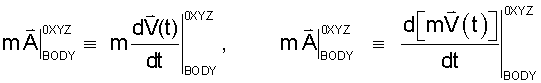
(6)6

Our next step is simply to substitute the extreme right side of (6) into the left side of (4).

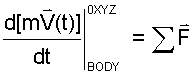
(7)7

The above form Newton's Second Law of Motion contains momentum, his axiomatic "quantity of motion," explicitly. Forces are physical "constructs" or reasons for change of momentum. Notice that when ΣF = 0, Equation (7) becomes Newton's First Law of Motion.

Newton's perspective, the "subjects of his Laws," is made clear upon understanding the axioms he set down as basis. The first axiom established the existence of a "quantity of matter." No proof is required in an axiomatic method. Mass (a measurable scalar property of matter) is possessed by a body and is of importance in its motion. A second axiom Newton called "quantity of motion." Today we call that quantity of Newton's, momentum. Momentum is less easy to quantify that is mass. Momentum is the produce to mass times its velocity define than mass. Newton was obliged to use vectors to quantify position (relative position, he realized) as a prerequisite idea. Space needed to be quantified - a vector space. Change of position in time begat velocity. which required vector calculus. Velocity is the derivative ov the vector, position. This momentum is the principle and second idea of his laws of motion.

The form of Newton's Laws of Motion selected for this writing is arranged in accord with Newton's axiomatic approach. Calculus is made explicit; the derivative of body momentum is placed prominently, left of equality. Subscripts of the derivative to identify the system (body for now). Superscripts to the derivative to identify the vector space. Finally ΣF replaces F right of equality in s differential equation, where non-homogeneous terms belongs. Vectors... all properly notated. Phew! Also we need the initial conditions written near the differential equation.

Mathematical notation says this better than words.

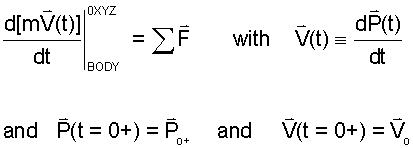
(8)8

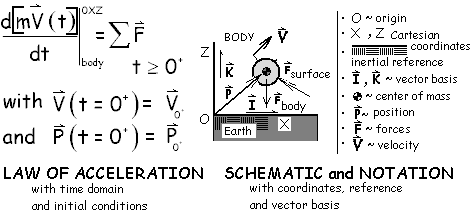
In use, Newton's equation is accompanied by a sketch of the physical situation. The sketch below is over- complete. In any application such completeness is rarely needed. However the sketch puts a picture to all of the ideas brought together.

There is a highly compelling reason students should use the differential equation form of Newton's Laws of Motion. In later engineering study you will find the mass equation, momentum equation and energy equation for a body have the same form. All three physical statements are first order differential equations (with their respective initial conditions). The skills and understanding gained in solving any of them are the same skills needed to solve and understand the others. All three equations take the same perspective - system.

(9)9

By physics, the center equation (which includes the idea "f = ma") would be written "f = ma." The left and right equations are engineering rate form expressions that account for changes of mass and energy of a BODY as system, respectively.

Further Reason: In closing, even if Newton did not use the formulation of Equation (7), we should use it. It does everything "f = ma" does and it expresses the mathematical meaning of Newton's Laws better. Since 1687 science and engineering has used the form more and more. For example, to describe expansion of the universe. The mean distance " l " between conserved cosmological particles is increasing with time. The mathematical statement of the rate of increase of this mean distance is written as:

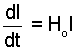(10)

This also, is a first-order differential equation!

Newton studied Cosmology and he used the rate form.

Further proof of the power of the first order differential equation, read about the mathematics of the Lotka-Volterra Predator-Prey Equations.

Momentum also is "what matter has" until it changes. Far better (than "f = ma" ) for engineering is the system property perspective of momentum, written (for a BODY) as before:

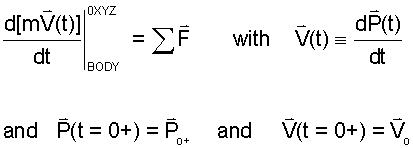
(11)11

In this writing, "BODY" (uppercase) is used rather than "body" to emphasize that Newton studied the former which is a very special, isolated, perspective of the latter. Newton used mathematical and physical analysis to study physical reality. He sought to discover the methods and perspectives of study that would reveal the secrets of nature. His approach, which we use today, might well be called Newton's Analytic Method." In any analysis, his very first step was a complete mental and mathematical identification and extraction of the body from its space to become a BODY for analysis.

While there are three laws, by strict way of usage Newton's Second Law is sufficient. The First Law represents its truth but the statement of Second Law, for the case of "zero net Force" is equivalent (there is reason to view the First Law as a special case of the Second Law). Newton's Third Law is different altogether. It does not apply to a BODY. Rather it applies as a condition or rule regarding the inter-dependence of forces within systems of two or more BODIES. Thus, in application, Newton's Laws of Motion are competently represented by his Second Law of Motion (2'nd Law). In high school, "force equals mass times acceleration" is 2'nd Law mantra.

The idea, "Force," was created (prior to Newton) as a means or "strategem of use" in understanding motion. Two types were understood as: those "acting at the surface" and another "acting from a distance" over the mass of the BODY, calles gravity. Force, is a "construct."

As a recent and excellent example see Richard Fitzpatrick's Newtonian Dynamics, page 9 (briefly paraphrased here).

1.10 About  f = ma

In 1687 Newton published his famous Three Laws of Motion. Every two or so years thereafter, some scholar, physicist or engineer has published an article or text chapter in which "What Newton Meant," is explained. That debate continues. A recent, representative writing (which I paraphrase here) is Richard Fitzpatrick's Newtonian Dynamics.

In presenting Newton's 2'nd law to students, HS physics avoids the vectors and calculus Newton invented and used. Most physics texts reduce Newton's understandings of motion to an algebraic equation. Three equational notations used in physics texts are:

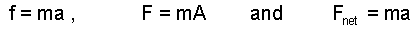(1)

Premise presently unwritted!