THERMO Spoken Here! ~ J. Pohl © ( A2380~9/15)

1.07 Mass Equation: BODY

Although Newton studied physical reality, he studied "simplified" to the vantage of a model. The system of Newton's studies was a constant physical mass, the BODY. It is unlikely that Newton expressed the constancy of the mass as a first order differential equation. Here we do just that. Not to be fancy but to set a sound beginning for the property equations that will be developed. Therefore we write the mass equation of a BODY with in its equivalent mathematical forms.

Rate Form	Differential Form	Increment Form

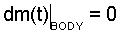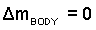Explanation of the Equation Forms: As we will see in our studies, different mathematical forms of equations are used. The steps about to be taken are the very basic steps of classical calculus. Beginning with the property of a BODY, its mass, steps of symbolic mathematics are used to express a "rate form" differential equation that describes the change of the mass with time. For mass of a BODY, this is a simplistic task. However, this derivation is fundamental. The identically same process of thinking is used with momentum and energy and for each of the system types, in succession.

Although the mass of a BODY is constant, we can represent it as a function of time.

(1) 1

Now consider a time, t = t*, and a time thereafter notated as, t = t* + Δt. The notation, Δt represents a usually short increment of time (some authors use lowercase as, δt). The masses of the BODY for these two times can be written as:

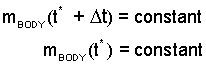
(2)2

The equation that represents mass of the BODY at time, t* + Δt is written above the equation representing mass at t* because we intend to perform a subtraction of the equations.

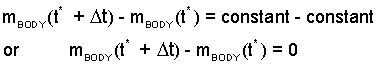
(3) 3

The lower expression of (3) is called the "difference" of mass of a BODY (Some write this as Δm_BODY = 0). The distinction of the masses occurs over the change or increment of time, Δt. We divide (3) by Δt (below left) and since 0/Δt = 0, we have below right.

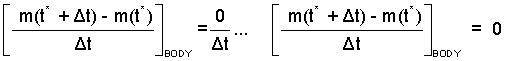
(4) 4

Next we take the limit of (4) as Δt becomes smaller to the point that it vanishes, becomes zero. That mathematical idea is written below left. To the right of the limit, it is written again, that is with another notation. The math symbol, ≡ means "is equivalent to."

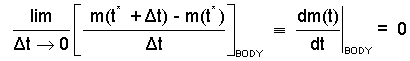(5) 5

The rate form equation, (5), is general. To apply the equation to a physical circumstance requires an additional piece of information called the initial condition. The mass at the initiation of observation must be known. Our result (as commonly used) is written above right. We will use it to obtain the other forms.

Equation Forms: The form we have derived (Eqn-5) is called the "rate form." It is the "d/dt" that operates on mass that identifies the equation as having this form.

Equation - (rate form):

(6) 6

The next form is obtained simply by "separation of variables." When the variables of the rate form equation are separated, the result is the differential form.

Equation - (differential form):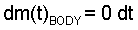

(7) 7

The path to the difference form begins at the rate form. Separate variables, then integrate.

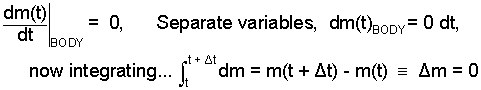(8) 8

The above line shows the path to the increment form equation.

Equation - (increment form):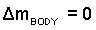

(9) 9